From Tensegrity to Flextegrity
(aka, “How in the heck did you ever think this up??”)
Inventors with strikingly novel concepts are often asked, “How in the world did you ever come up with that idea?”. My experience as author and creator of Flextegrity’s new technology has been no exception. However, my surprise is not that these ideas emerged now in the evolution of structural materials, but in the fact that it took so long!
An enormously significant leap forward in structural design was, in fact, Buckminster Fuller’s discovery and development of the geodesic dome in the early 1950’s. In parallel flowed Fuller’s and Kenneth Snelson’s co-invention of ‘tensegrities’ with groundbreaking explorations confirming the theoretical ‘discreteness’ of tensile and compressive properties of structure.
Photo: Onderwijsgek
Tensegrity is a contraction of two terms, tensile + integrity, and refers specifically to structures which exhibit the principles of discontinuous compression and continuous tension. Over the years, depending on with whom you talk, the term as has been narrowly applied to structures that use straight slender compression elements in a tensile (typically wire) array. I am not an adherent to this limitation as I think it is overly arbitrary and limiting, and it does not address the issue of omni-axial loading.
Given the intrinsic and clear advantages of tensegrity structures (there are at best just a few multi-purpose, 3-dimensional structural configurations possessing equal promise), it is perhaps surprising that little organized or aggressive research into potential commercial applications has been pursued in the decades following. But given the human tendency to cling to the familiar, the technological inertia must be attributed largely to embedded resistance to changes in existing construction methodologies, not to the known integrity and usefulness of lattice networks and other tensile designs.
But please indulge me in a brief personal digression: I begin by confessing my own affection for tensegrities. I have built many models over many years. They possess an ethereal and resonant equilibrium that lures the observer into a false sense of order and structure.
A parallel verisimilitude descends on those who study concepts of crystalline ‘close packing’ and geodesic geometries. Combined, the resulting orderliness of space seems nearly magical in its precise dimensions and simultaneously tempts us with suggestions for a universal order. We can easily persuade ourselves that we have arrived at the cosmological simplicity of implicit structural order and may dare to construct a ‘self’ from its ordered parts. A ‘Natural Theology’ – arguably better than the many it replaced – but an act of faith nevertheless.
I write these words reflecting on my own world with its constructions of lumpy matter representing gravitational compressive forces and infinite currents of electromagnetic movements tied together in Fuller’s knotted integrity. In my past paradigm, I was tensegrity. And, just as we are sometimes liberated but many times imprisoned by the mythology, symbols and eloquence of preceding generations, I confess to have fallen into the trap of having found order in chaos while entirely and happily overlooking the deficiencies of the thesis!
As often as I allowed these thought processes to describe the textural qualities of life as ‘discrete’, ‘linear’ and in tension and then to make diagrammatic representation with vectors and points, seldom did the paradigm coincide with my own messy and more complex experiences of ‘entanglement’ and ‘growth’— not easily assembled from struts and wire or depicted in diagrams. The challenge of ‘diffeomorphism invariance’ had no home in my world. This thesis fundamentally states that there are no points in space, simply locations at which events take place. My ‘Bohr atom’ model fell in tatters. No points, no lines, no structure!
To be rigorously honest and to be engaged in the process of authentic discovery, one must journey without charts or compass and not follow the worn paths that steer us unconsciously towards some distant preordained conclusion. Somewhere along that path I was compelled to find a different route and I arrived at new conclusions about the very concept of discontinuous compression and continuous tension in general, and about tensegrities specifically. First and foremost is that tensegrities don’t have a biological analog! They are not inherently anticipatory or comprehensive.
Tensegrities as structures are coherent by necessity. They are fully formed—that is, you must define the structure in its entirety before you can build it. That is true when building tensegrities of the regular polyhedra and in any artistic expressions of the structure. Each strut, each tension member length must be mathematically predetermined. Changing the relative size of the cells is a problematic exercise as you cannot increase or decrease the unit size without premeditation of the outcome.

Photo: niko photos
Biological systems, on the other hand, are not prescriptive in ‘knowing’ in advance exactly what the mature tree will look like. The genetic code for a tree doesn’t predict the tree—it is simply the building blocks necessary to build the tree. The tree ‘grows’ into its form.
Anticipatory and comprehensive materials and their organization contain the basic building blocks for replication and allow the spontaneous arrangement of parts without prior knowledge of the ‘whole.’ Atoms form molecules and molecules organize into cells and so on. The atoms have no knowledge of the cells and are surprisingly indifferent to larger organizational patterns expressed in the cell.
Columnar struts are elegant, slender and are optimal shapes for carrying axial loads. Tensegrities are described as having omni-axial integrity in tension but they are quite unquestionably axial in compression. Because we live in a compression-dominant world, this constitutes a major flaw. If, for example, an external force is applied that is 45 degrees from the axis of the tensegrity’s column then it is being borne by a tension member. The tension member in turn is transmitting the non-axial loads to the compression column which is now subject to bending moments for which is not designed. The orthogonal organization of the compression members and the shape itself dictates less-than-optimal handling of forces other than those in the axial loading direction.
The problem is that loads in nature are rarely, if ever, purely axial. Biological structures respond to forces from many directions. For example a fish withstands tremendous pressures at great depths without collapsing. The forces on top are the same as on its soft belly. Natural structures must be designed to economically withstand omni-axial loading. An ordered patterned microcellular array can be optimized to withstand axial loading and bending moments both in tension and compression.
Tensegrities are not omni-extensible. When you construct one ‘cell’ defined as a coherent tensegrity unit—an octahedron, for example—you cannot attach the next cell without ‘dismembering’ one or more struts and affected tensile elements. If you take a simple octahedral tensegrity and snip a single wire, the integrity is completely lost. There is no intrinsic accommodation for growth. Nature on the other hand is inherently parsimonious in the manner in which it binds. It can’t constantly invent new ways to connect—in this case, wires (tension) and struts (compression.) These are complicated mathematical constructions.
A comprehensive building system anticipates the development of structural forces and morphs as it encloses space and responds to loads. If you have played with Lego’s, you know the elegant simplicity of a system of blocks of known sizes and a single connection design. There is one rule—A connects to B in one way—but no rigid blueprints. A child starts building (creating) and never knows where he or she might end up!
Tensegrities possess no means of reproduction. As a carpenter builds a house of wood by nailing, sawing, gluing and screwing so must a tensegrity be assembled into something from disparate parts that otherwise wouldn’t coexist. In order for a tensegrity cell to divide it would either need to possess either the entire code necessary for reproduction or allow tension and compression to ‘asexually’ pair.
A natural structural building system is self-replicating; each cellular element possesses its own integrity and the means to connect and shares the integrity of the whole. Self-replication requires the means to adapt, grow and morph. Much as stem cells differentiate into bones and muscles, so, too, do structures require local prescriptive advantages with global integrity. As Fuller was very fond of reflecting on how bubbles naturally form in the froth of the oceans, Nature doesn’t calculate pi in order to form a perfect sphere! Nor do cells.
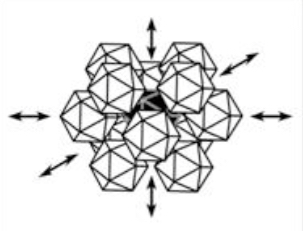
Tensegrities are elegant abstractions—they are not a theory that explains the nature of tension and compression. They are, by design, exclusive. Tensegrities don’t allow for serendipitous events, omissions, or transcription errors. Over the years I’ve developed the ‘ecosystem’ tensegrity model. An icosahedral tensegrity is a good example because of its complexity. The compression columns represent the higher mammals or fauna within the ecosystem. The entire structure represents the system in equilibrium. While you can remove wolves (a ‘strut’), for example, from an ecosystem it puts an imbalance and strain on the rest of the system; remove too many elements from the system and it collapses.
Tensegrities explain one network of interconnection between tension and compression but they do not explain the whole, the whole being greater than the sum of its parts. It must, in order to be synergistic. Fuller himself noted that “compression is multi-axial and omni-topologically co-exists with tension in infinitely omni-extensible arrays”! That idea began to gnaw at me—what paradigm was necessary in order to build such an array?—and eventually led to my evolution to ‘flextegrities.’
In summary I’ve described the deficiencies I’ve observed in tensegrities as a biological model. They are inherently coherent and closed. They are neither omni-extensible nor optimized for omni-axial loading. Tensegrities are not self-replicating and hence they can never transcend their own inherent cellular limitation. I have mentioned many of the properties of the Flextegrity material. Flextegrity’s arrays are superior in almost every characteristic important to designers and engineers, the most compelling of which is that materials should mimic nature’s wisdom. I am not suggesting designing buildings using literal models of natural macrostructures, but by using nature’s own microstructural example to provide improvements in strength-to-weight ratio, porosity, omni-axial loading and many more necessary characteristics.