ICOSAHEDRON COMPRESSION STUDY

Introduction and Background:

The project started with an initial compression model of a symmetric icosahedron array modeled after a sheet steel structure. Analysis results indicated that the compressive load carrying capacity of the structure is dominated by the connecting ligaments. That is, under compressive load, the connectors want to buckle and irreversibly deform. Once the icosahedrons touch, the load bearing capacity of the structure is significantly increased. Subsequent to these results and additional engineering meetings, a spreadsheet was developed that allowed the direct calculation of the bending load carrying capacity of the structure. The theory behind this spreadsheet is that the load is carried by the connecting ligaments that are perpendicular to the applied load and run in the direction parallel to the bending axis. In brief, in the orthogonal array of connectors, only one line or one direction of connectors carry load. The other 2/3 thirds of the connectors act to hold the structure together and allow it to behave as a “beam” like structure.

Some discussions were had whether or not the structure behaves like a cable or a beam, but at the end,it was reasoned that it behaves like a beam since it can bear transverse load while simply-supported at itsends without an axial constraint. In contrast, a cable requires axial constraint to bear a transverse load.

Lastly, a study was done on the compressive load carrying capacity of just the icosahedron.

Watch the load carrying capabilities of a single icosahedron under compression

This report addresses this study. The load capacity is defined as that load the structure can carry in a linear, elastic fashion. To be linear elastic implies that upon release of the load the structure will elastically return to its original shape.

Modeling Details:

A simple icosahedron having an edge length of 1.0” was used. The icosahedron was assumed to be in a densely packed array and that the deformation behavior could be modeled as if it was squeezed between two large plates. This assumption allowsone to understand the basic compressive load carrying capacity of the icosahedron for design purposes.

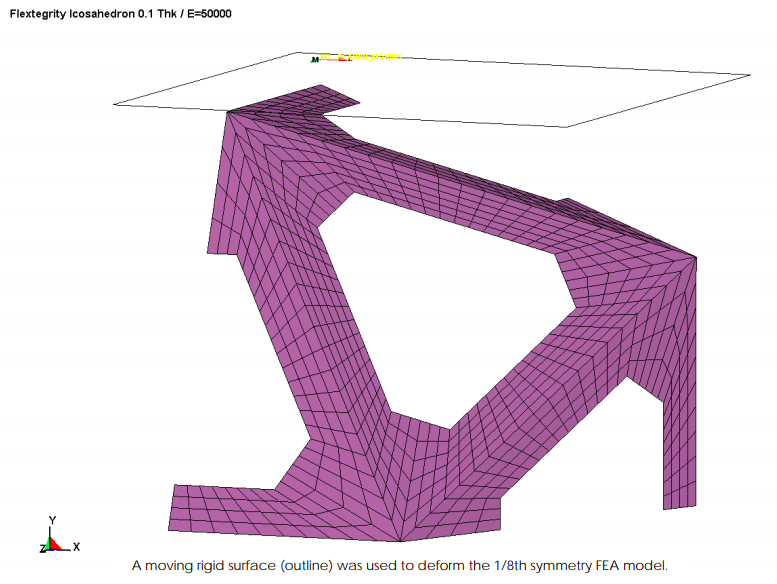
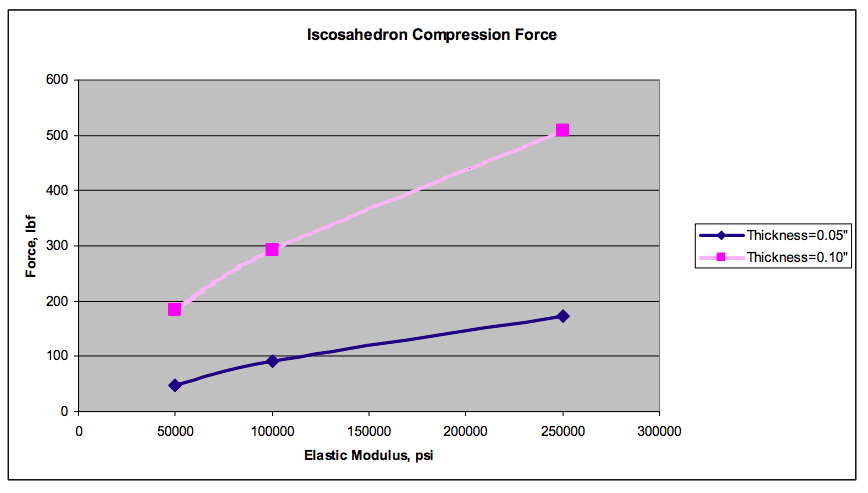
The finite element model was based on a 1/8th symmetric section of the icosahedron and loaded via a flat plate pushing into the top of the icosahedron. Charts are provided showing the load versus deformation behavior. Two thickness and three elastic moduli were investigated, yielding six data points. The following page shows a graph of these results.

Summary:

Compressive failure of the icosahedron is by buckling. For the thick (0.10” thick) icosahedron’s, the buckling is along the top member while for the thinner (0.05”) icosahedrons, the side members buckle. The results follow a logical trend with increasing thickness and elastic modulus providing superior compressive strength.

Check out the behavior of an open-faced icosahedron under compression, and read the full study here >>>